At the heart of mathematical transformations lies the codomain, a concept that shapes the very nature of how functions operate. This discourse delves into the intricacies of the codomain of the transformation X, uncovering its definition, types, properties, and real-world applications.
Prepare to embark on an enlightening journey that will unravel the mysteries of this fundamental mathematical concept.
The codomain, a set of potential output values, plays a pivotal role in determining the behavior and characteristics of a transformation. By examining the relationship between the codomain and the range, we gain insights into the injectivity, surjectivity, and bijectivity of transformations.
Codomain Definition and Concept
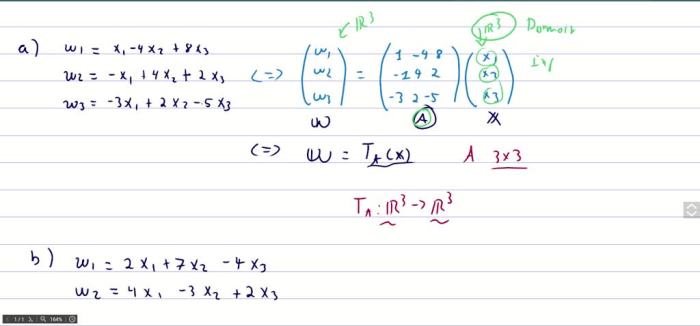
In mathematics, the codomain of a transformation is the set of all possible output values. It is typically denoted by the letter Y. The domain, on the other hand, is the set of all possible input values, denoted by the letter X.
The transformation itself is a function that maps each element of the domain to an element of the codomain.
The codomain is an important concept in transformation theory because it helps to define the range of the transformation. The range is the set of all output values that are actually produced by the transformation. In other words, the range is a subset of the codomain.
For example, consider the transformation f(x) = x^2. The domain of this transformation is the set of all real numbers, and the codomain is also the set of all real numbers. However, the range of this transformation is the set of all non-negative real numbers, because squaring a real number always produces a non-negative result.
Types of Codomains
There are different types of codomains, depending on the nature of the transformation. Some of the most common types of codomains include:
- Sets: A set is a collection of distinct objects. The codomain of a transformation can be a set if the transformation produces a unique output value for each input value.
- Ranges: A range is a subset of a set. The codomain of a transformation can be a range if the transformation does not produce all possible output values.
- Spaces: A space is a set that is equipped with a topology. The codomain of a transformation can be a space if the transformation is continuous.
The type of codomain that is used for a particular transformation depends on the specific properties of the transformation.
Codomain and Transformation Properties
The codomain of a transformation can affect the properties of the transformation. For example, the codomain can affect whether the transformation is injective, surjective, or bijective.
- Injective: A transformation is injective if it produces a unique output value for each input value. In other words, the transformation does not map any two distinct input values to the same output value.
- Surjective: A transformation is surjective if it produces all possible output values. In other words, the range of the transformation is equal to the codomain.
- Bijective: A transformation is bijective if it is both injective and surjective. In other words, the transformation maps each element of the domain to a unique element of the codomain, and it produces all possible output values.
The codomain of a transformation can also affect the continuity of the transformation. A transformation is continuous if it produces small changes in the output value for small changes in the input value.
Codomain in Applications: The Codomain Of The Transformation X

The codomain of a transformation plays an important role in many real-world applications. For example, the codomain of a transformation can be used to:
- Determine the range of the transformation.
- Determine whether the transformation is injective, surjective, or bijective.
- Determine the continuity of the transformation.
- Solve equations and inequalities involving the transformation.
- Model real-world phenomena using transformations.
The codomain of a transformation is a fundamental concept in transformation theory. It is used to define the range of the transformation, to determine the properties of the transformation, and to solve equations and inequalities involving the transformation.
FAQ Summary
What is the purpose of the codomain in a transformation?
The codomain defines the set of all possible output values that the transformation can produce, providing a boundary for the range of the transformation.
How does the codomain affect the injectivity of a transformation?
The codomain plays a crucial role in determining injectivity. If the codomain is too small, it can restrict the transformation’s ability to map distinct input values to distinct output values, potentially compromising injectivity.
Can the codomain be different from the range of a transformation?
Yes, the codomain can be different from the range. The range is a subset of the codomain that contains only the actual output values produced by the transformation, while the codomain encompasses all possible output values.